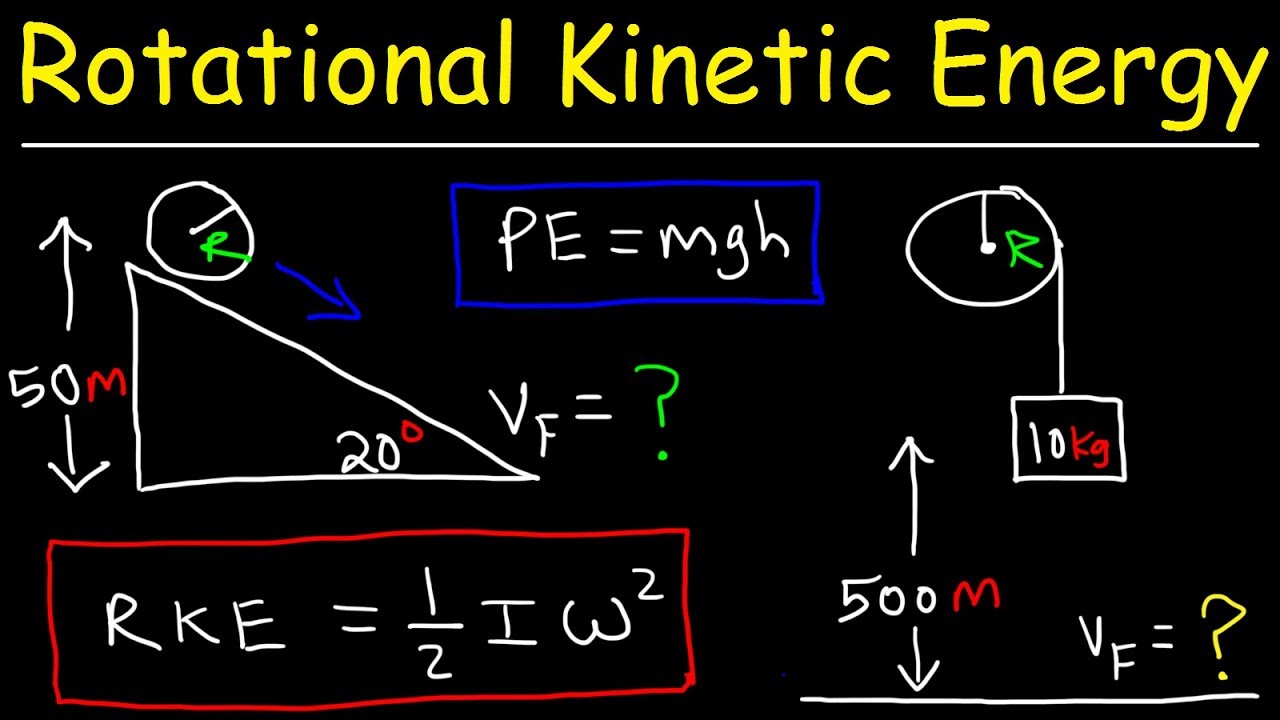
Unveiling the Spin: Exploring AP Physics 1 Rotational Motion

Imagine a spinning top, a whirling bicycle wheel, or the Earth itself rotating on its axis. These captivating displays of motion, seemingly simple yet profoundly complex, lie at the heart of rotational motion, a cornerstone of AP Physics 1. This intricate dance of objects around an axis presents a fascinating challenge for students, demanding a shift in perspective from the familiar world of linear motion.
Understanding rotational motion isn't merely about memorizing equations; it's about developing an intuitive grasp of how torque, angular momentum, and moment of inertia intertwine to govern the behavior of spinning objects. This conceptual understanding is crucial for tackling the diverse array of problems encountered in AP Physics 1, from calculating the angular acceleration of a rolling cylinder to analyzing the stability of a rotating gyroscope.
The study of rotational dynamics has its roots in classical mechanics, dating back to the pioneering work of scientists like Galileo and Newton. Their observations and mathematical formulations laid the groundwork for our modern understanding of how forces influence rotational motion. The importance of rotational motion extends far beyond the classroom. It's fundamental to countless applications in engineering, from designing efficient engines and turbines to understanding the movements of celestial bodies.
One of the main challenges students face with AP Physics 1 rotational motion problems lies in applying the concepts to real-world scenarios. Translating a word problem into the appropriate equations and variables requires careful analysis and a deep understanding of the underlying principles. This is where practice becomes paramount. Working through a variety of problems, from simple to complex, is essential for developing the problem-solving skills needed to succeed on the AP exam.
The concepts in rotational motion are analogous to those in linear motion, but with key differences. For instance, instead of force, we have torque, the rotational equivalent that causes angular acceleration. Similarly, moment of inertia takes the place of mass, representing an object's resistance to changes in its rotational motion. These parallels can be helpful in understanding the concepts but also require careful attention to the distinctions between linear and rotational quantities.
One benefit of studying rotational motion is developing critical thinking and problem-solving skills. The complex nature of rotational problems requires students to analyze situations, identify relevant variables, and apply appropriate equations, fostering analytical abilities. Furthermore, the study of rotational motion provides a deeper understanding of the physical world around us, from the motion of everyday objects to the behavior of complex systems like planetary orbits. This knowledge can be valuable in a wide range of fields, from engineering and physics to astronomy and geophysics. Finally, mastering rotational motion in AP Physics 1 lays a strong foundation for future studies in more advanced physics courses.
A successful approach to tackling rotational motion problems involves a systematic process: First, carefully read and understand the problem statement, identifying the given information and the unknown variables. Second, draw a free-body diagram showing all the forces and torques acting on the object. Third, choose the appropriate equations based on the problem's specifics, and solve for the unknowns. Regular practice with diverse problem sets is crucial for refining this process.
Frequently Asked Questions about AP Physics 1 Rotational Motion Problems:
1. What is torque? Torque is the rotational analog of force, causing an object to rotate around an axis.
2. What is moment of inertia? Moment of inertia represents an object's resistance to changes in its rotational motion.
3. How is angular acceleration calculated? Angular acceleration is the rate of change of angular velocity.
4. What is angular momentum? Angular momentum is the rotational analog of linear momentum.
5. What is the relationship between torque and angular acceleration? Torque is equal to the moment of inertia multiplied by the angular acceleration.
6. How do I solve rotational motion problems involving rolling without slipping? In these cases, the linear velocity of the center of mass is related to the angular velocity.
7. What are some common mistakes to avoid in rotational motion problems? Common errors include confusing linear and rotational quantities, neglecting friction, and not considering the direction of rotation.
8. Where can I find more practice problems? Numerous resources are available online and in textbooks specifically designed for AP Physics 1.
One tip for solving rotational motion problems is to always consider the direction of rotation, using the right-hand rule to determine the direction of angular quantities. Another trick is to simplify complex systems by breaking them down into smaller, more manageable parts.
In conclusion, the study of rotational motion in AP Physics 1 presents a unique and challenging, yet rewarding, exploration into the fundamental laws governing the universe. Mastering this intricate dance of spinning objects, from the smallest particles to the largest celestial bodies, not only equips students with essential problem-solving skills but also fosters a deeper appreciation for the elegant interplay of forces and motion that shape our world. Embracing the challenge of rotational motion problems, with diligent practice and a curious mind, opens the door to a richer understanding of the physical world and paves the way for future explorations in science and engineering. So, dive in, explore, and let the spin begin!
Unlocking the power of farrow and ball great white
The ultimate guide for crossword enthusiasts
Unlocking the power of navy blue your ultimate color pairing guide